
Wilkes published a paper in 1953 discussing relative addressing to facilitate the use of subroutines.
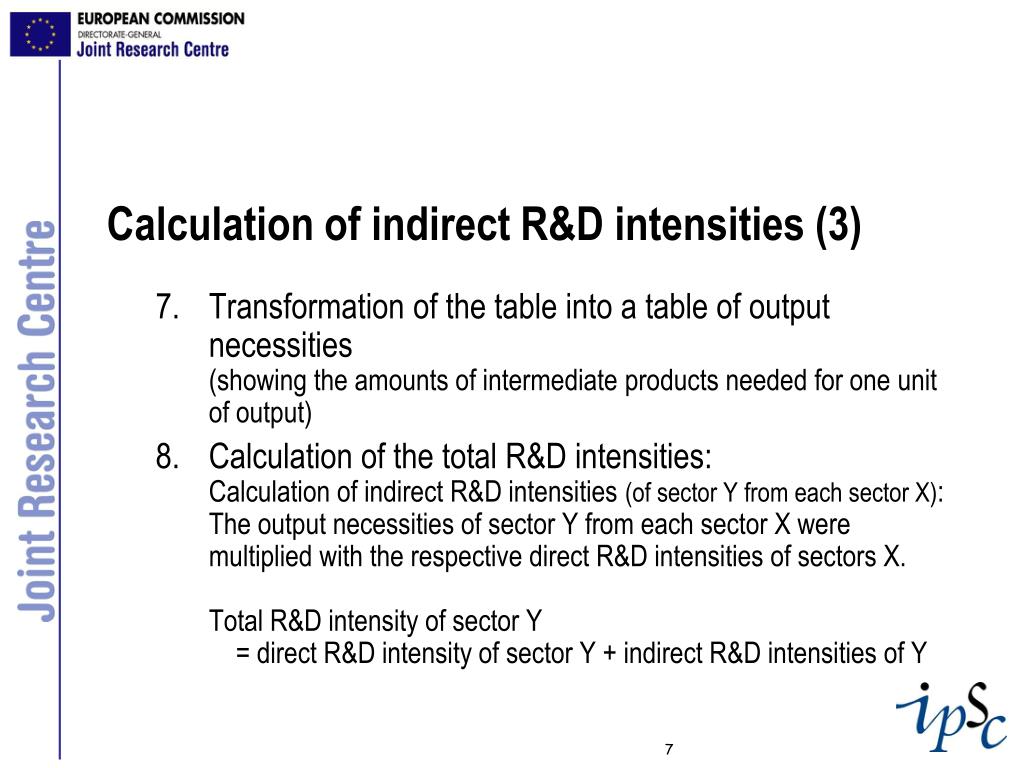
#LEVEL OF INDIRECTION HOW TO#
Along with Maurice Wilkes and Stanley Gill, he is credited with the invention around 1951 of the subroutine (which they referred to as the closed subroutine), and gave the first explanation of how to design software libraries as a result, the jump to subroutine instruction was often called a Wheeler Jump. Wheeler's contributions to the field included work on the Electronic delay storage automatic calculator (EDSAC) in the 1950s and the Burrows–Wheeler transform (published 1994).
He was awarded the world's first PhD in computer science in 1951. In 1945 he gained a scholarship to study the Cambridge Mathematical Tripos at Trinity College, Cambridge, graduating in 1948. His education was disrupted by World War II, and he completed his sixth form studies at Hanley High School. He was educated at a local primary school in Birmingham and then went on to King Edward VI Camp Hill School after winning a scholarship in 1938. But one ought to make a special effort to see that both ways of approach to the solution of the problem be laid open for thus not only is one solution greatly strengthened by the other, but, more than that, from the agreement between the two solutions we secure the very highest satisfaction.Wheeler was born in Birmingham, England, the second of the three children of (Agnes) Marjorie, née Gudgeon, and Arthur Wheeler, a press tool maker, engineer, and proprietor of a small shopfitting firm. Of course, when the effective causes are too obscure, but the final causes are more readily ascertained, the problem is commonly solved by the indirect method on the contrary, however, the direct method is employed whenever it is possible to determine the effect from the effective causes.
Since, therefore, two methods of studying effects in Nature lie open to us, one by means of effective causes, which is commonly called the direct method, the other by means of final causes, the mathematician uses each with equal success. Now there exist on every hand such notable instances of this fact, that, in order to prove its truth, we have no need at all of a number of examples nay rather one's task should be this, namely, in any field of Natural Science whatsoever to study that quantity which takes on a maximum or a minimum value, an occupation that seems to belong to philosophy rather than to mathematics. Wherefore there is absolutely no doubt that every effect in the universe can be explained as satisfactorily from final causes, by the aid of the method of maxima and minima, as it can from the effective causes themselves. For since the fabric of the universe is most perfect, and is the work of a most wise Creator, nothing whatsoever takes place in the universe in which some relation of maximum and minimum does not appear. Introduction to De Curvis Elasticis, Additamentum I to his Methodus Inveniendi Lineas Curvas Maximi Minimive Proprietate Gaudentes 1744 translated on pg10-11, "Leonhard Euler's Elastic Curves", Oldfather et al 1933Ĭontext: All the greatest mathematicians have long since recognized that the method presented in this book is not only extremely useful in analysis, but that it also contributes greatly to the solution of physical problems.


 0 kommentar(er)
0 kommentar(er)
